@reasoningmind I agree. But the best of us cannot always solve problems. Trick is to learn how to stay upbeat. Teachers job IMO #mathchat

x

Of course, by “us” here I mean the human race, and not any one particular individual. If problem solving is seen as a race or a competition, then yes, of course, there will be many problems that will defeat even the most talented students, and they may well become disheartened. Less mathematically able students can become disheartened a lot earlier.

Probably the most upbeat mathematical problem solver on this planet is Terry Tao. Chances are no one has ever been a better mathematical problem solver than Terry. He tackles some of the deepest and toughest mathematical problems there are, and manages to stay very upbeat about it all,  so there’s something to be learned from him about a positive attitude to problem solving and to mathematics in general. Terry wrote a helpful blog post “Solving mathematical problems“. He recommends playing with a problem:

“I find that “playing” with a problem, even after you have solved it, is very helpful for understanding the underlying mechanism of the solution better. For instance, one can try removing some hypotheses, or trying to prove a stronger conclusion.”

He also strongly advises that you ask yourself dumb questions – and answer them!

You may so it’s all very well for Terry Tao to be positive about mathematical problems: he’s shown a remarkable ability to solve hard mathematical problems since he was very young. That’s not how it is for me or for my students.

Ed Burger writes: “… my math teacher taught me how to ask questions and not give in until I was able to understand. My math teacher taught me how to look at the world and see it in a crystal focus. My math teacher taught me how to think and create.”

So how can mathematics teachers help students feel positive and upbeat about solving mathematical problems – from simple to hard? How can, as ReasoningMind suggests, even the best mathematics teachers overcome the dispirited feeling students get when they cannot solve mathematical problems?

I have a few suggestions to offer – only a few – but I hope they may go some way to helping classroom teachers work to keep an upbeat spirit for all students in their mathematics classrooms.

Ask yourself dumb questions – and answer them! (Terry Tao’s advice)

This is about the simplest and most useful piece of advice I know for keeping upbeat about mathematical problems. Here’s a good way to implement it. Start a diary for a problem. Use an exercise book, or a word document on a computer, in which a student records what they found each day they worked on the problem; a summary of calculations they did and conclusions they reached. The next time the student begins work on the problem read the previous diary entry and get them to ask the simplest dumbest question to which they do not yet know the answer – and set about answering it! This way, progress becomes palpable.

This assumes that the problems students are working on will not necessarily be expected to be completed in a single class period. For harder problems, limiting students’ working time to a single class is highly likely to lead to frustration, depression and a general air of being dispirited. Not good! Keep the problems that are expected to be completed in a single class session or for a set homework period, to routine problems.

Reduce cognitive load (John Sweller’s advice)

I wrote in an earlier post about my conversion to John Sweller’s viewpoint on problem solving. This is about reducing the demands on students’ working memory.  Teachers of mathematics need to think about and analyze the problems they set for students to see – as a best estimate – how certain problems might be stressing working memory of their students. Teachers need to instruct students in ways of reducing cognitive load – the demand on working memory made by certain problems.  When most problems a student tackles stress that student’s working memory beyond its capacity the student will soon give up, feeling defeated. The teaching issue is that this feeling of defeat never had to happen if the teacher had instructed students in ways of reducing the load on working memory.

The problem might be really, really hard

Some years ago my colleague Elena Steencken at Rutgers came into my office excited to tell me how the students and teachers in her group had found a beautiful pattern for a counting problem. I cannot remember the exact formulation of the problem but I do recall what happened next. I must have frowned because Elena said: “Uh, oh! The look on your face!” I asked what she meant, and she said I looked worried. Well, I was was worried. I thought about the problem later that day and realized it was a reformulation of the problem of counting self-avoiding walks of a given length.

This is a famous, very, very difficult problem in mathematics. It is very unlikely that a group of teachers and students would solve it in an hour or two, and it was certainly not true that Elena’s simple algebraic formula was correct. I told her so the next day, and she said they had tested it for various cases. I asked her to test it for the next case where I knew the answer had been worked out in detail: her formula did not agree with the known answer!

The moral: really, really hard problems are lurking everywhere in mathematics. You may have stumbled across one. Check your answers and then ask a professional mathematician if they know, or knows someone who knows,  something about the problem. This is not failure – it’s real authentic learning. It is discovering facts about the nature of the mathematical landscape – mathematical reality as distinct from textbook knowledge. It’s fun, it’s exciting, and anyone can do it. But beware that your problem might be genuinely really hard. Understanding why develops mathematical maturity.

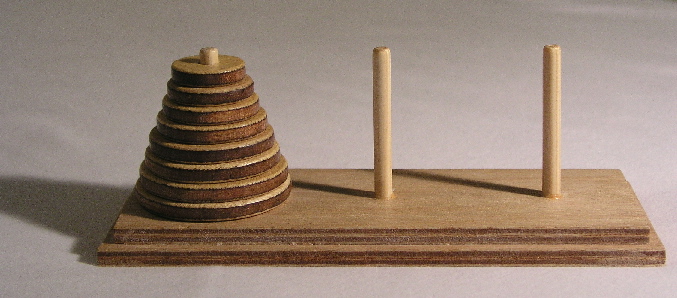Here’s an example of how easy it is to misunderstand the difficulty of a problem. The Tower of Hanoi problem is a well-known and often used example in problem solving for upper elementary and middle school students. Often enough students will come up with a recursive solution to the tower of Hanoi problem. What have they solved? They have shown, essentially that with 3 pegs and n disks on 1 peg it is possible to shift all the disks, following the rules of moving only one disk at a time and never putting a larger disk on a smaller one, to another peg in moves. What they have not done is to show that this is the shortest number of moves possible: that takes a different, more subtle argument.

Now what happens when we move to the 4-peg version of the Tower of Hanoi? Many people suspect, but to this day no one knows, the correct answer for the shortest numbers of moves to complete the puzzle. That is hard mathematics, arising from a simple puzzle.