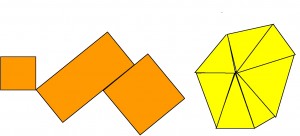
Why does a triangle have an area?
Posted by: Gary Ernest Davis on: February 23, 2011
In a lovely series of videos James Tanton clarifies the formula for the area of a plane triangle.
Starting from some simple postulates, namely:
- area is additive over finitely many parts that do not overlap;
- congruent figures have equal area;
- a rectangle of length
and width
has area
James shows that, if a triangle has an area then the area of the triangle must be half the length of the base of the triangle multiplied by its height.
Unfortunately there is nothing in the assumptions, or the demonstration, that allows us to show that a triangle HAS an area.
We have postulated rectangles have areas, and we have postulated that we can add up areas provided we do not overlap figures, and that we can move figures with areas around by congruences so as to have figures with the same area.
All we can get from (1)-(3) is figures that are non-overlapping unions of a finite number of congruent transformations of rectangles.
Triangles are not such beasts.
So we have a problem in asserting that a triangle even HAS an area.
Of course we can add it in as a postulate:
4. Every triangle has an area.
Then James’s argument in the videos is perfectly valid, and establishes a formula for the area of a triangle.
Well what’s the fuss you may ask?
Once we have triangles having area, and knowing a formula for their area, we can surely split all polygons into non-overlapping triangles and show that polygons have areas, and obtain a formula for that area.
Indeed we can.
But now, from the perspective of general polygons we see that triangles are somehow more fundamental than squares or rectangles: we can build polygons out of non-overlapping triangles, but we cannot generally build them out of non-overlapping congruent transformations of rectangles.
So the assumptions (1)-(4) are a little redundant, and maybe we should have assumed:
(A) area is additive over finitely many parts that do not overlap;
(B) congruent figures have equal area;
(C) a triangle of base length and height
has area
Now it’s straightforward to see that a rectangle has an area and that area is its length times its width.
The point is, as James says several times in the videos, area, even of plane figures, is not such a simple notion as we might at first think.
Area as “amount of stuff” just does not cut it.
Once we get to odd regions, such as circular regions we are forced to abandon the assumption of only a finite number of parts in assumption (1).
Once we abandon that assumption and allow infinitely many non-overlapping parts then we CAN prove from (1)-(3) that every triangle HAS an area, just as we can prove that every circular region has an area.
A notion of area based on finite additivity – as in assumption (1) – is very restricted, and one has to make ad hoc assumptions as to what plane figures actually HAVE an area.
Infinite additivity (strictly speaking, countably-infinite additivity) allows us to do much more.
So let me say again, as James Tanton says in the videos: area is NOT straightforward, it is NOT simply “amount of stuff”, it has more to do with decomposition into parts known to have area than anything else, and passing to plane figures such as circular regions forces us into thinking about decompositions into infinitely-many parts.
And, just for good measure (if you will pardon the pun) triangles are probably more fundamental plane figures than are rectangles.
Postscript
As Alexander Bogomolny (@CutTheKNotMath) points out, if one takes as the area of a triangle as a definition, one is obliged to show it does not matter which base, and which height.
Alex has a very nice post on area at CTK Insights: “Does Triangle Have Area?”
A challenge to mathematics teachers: can your class become a community of practice?
Posted by: Gary Ernest Davis on: February 23, 2011
Jean Lave and Etienne Wenger wrote an influential book: Situated Learning: Legitimate Peripheral Participation (Learning in Doing: Social, Cognitive and Computational Perspectives).
As a budding mathematics educator this book had a big influence on me and, over the years since first reading it, I have often reflected how different a typical mathematics classroom is from a community of potters.
Potters get together to share techniques, to learn from each other, to discuss projects and progress, because they are interested in making pots, or other objects from clay.
Students in a mathematics class are, in the main, doing things they do not want to be doing, because someone told them they need to know it, and generally have to listen to large amounts of instruction to do those things they did not want to do in the first place.
There’s nothing terribly “legitimate” about most mathematics classrooms.
Could it be otherwise?
To a large extent a mathematics class is going to be focused on what the teacher thinks the class is about.
There are times when what the mathematics class is about is just doing mathematics.
For example I have taught an undergraduate class called “Mathematical Inquiry” and what that class is about, in my mind, is solving mathematical problems and looking as deep as we can into mathematical questions. Which questions we look into is a moot point – sometimes questions i come up with (because I’m more experienced, and so the teacher) or sometimes questions the students come up with.
One of the courses my colleagues and I run is simply a workshop/seminar course in which students choose a topic in conjunction with an adviser and dig as deeply as they can into this topic over the course of a semester. That class runs more like a pottery session. Students are doing what they want to do, sharing progress with each other, learning from each other, and the more experience people in the class.
Currently I am teaching statistics and differential equations. I have written something about the differential equations class here. Despite that class being project based there is nothing “legitimate” about the class: most students would simply stop coming if I offered them a B grade right now. After Spring break I hope to focus on applied modeling, particularly on differential equation models for cancer growth. I am hoping this will engage the students, yet it still only makes the class interesting, not legitimate.
The statistics class is based on the book StatLabs by Deborah Nolan and Terry Speed (my former teacher).
I like this book a lot because it bases the learning of statistics on applied case studies, and helps students get a feel for what a mathematical scientist does.
After Spring break I want students to focus on their own data sets, so as to get more invested in the analysis. Maybe this will help the class become more legitimate.
Already 3 students are focusing on their own data: one is interested in how many steps different people take to walk a mile, another in autism and population rates in the various US states, and another in comparisons for quarterback completion rates.
So the statistics class is heading toward legitimacy, in my view.
But this is generally hard work, I find.
It’s not hard helping students learn the techniques they need to analyze data. What is hard is getting them to be interested in the first place: it takes time and effort to draw out their interests.
Form a “curriculum” point of view there is, of course, always the “danger” that some supposedly vital aspect of the curriculum will not be “covered”.
I say, as clearly as I can: screw the curriculum. I do not cover material. I engage with students in legitimate mathematical activity, through doing and participation, as best I can.
Most of the time that’s not good enough, but I’m going to keep trying.