Wikileaks Facebook fan statistics
Posted by: Gary Ernest Davis on: December 1, 2010
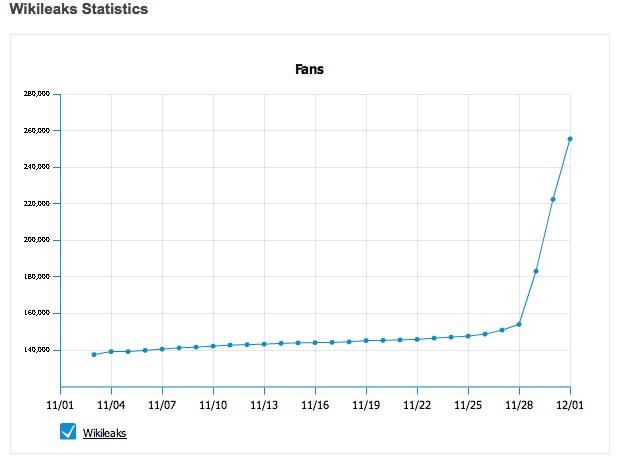
The chart below shows a sudden change in the growth rate of Facebook fans for Wikileaks:
When linear growth becomes exponential the underlying mechanism driving growth has changed.
Clearly something happened prior to, prescription or around, physician November 28 to change the model of fan acqusition to the Wikileaks Facebook page.
This is not just a change in growth: it is a change in the model of growth, from one of steady linear increments, to a model of exponential expansion.
Whether that growth flattens out or continues to grow exponentially for a while, time will tell.
The source for the chart is here.
How fast do the Fibonacci numbers grow?
Posted by: Gary Ernest Davis on: December 1, 2010
Fibonacci numbers
The sequence of Fibonacci numbers begins .
The defining feature of these numbers is that each is the sum of the preceding two:
.
We can write this defining property as a recurrence relation by naming the Fibonacci number
, starting from
.
So, .
The recurrence for the is then:
x
for
with
………………………..(1)
x
How rapidly do the Fibonacci numbers grow?
When we calculate a few of them we notice a curious thing about the ratio :
| 0 | 1 | |
| 1 | 1 | 1 |
| 2 | 2 | 2 |
| 3 | 3 | 1.5 |
| 4 | 5 | 1.666666667 |
| 5 | 8 | 1.6 |
| 6 | 13 | 1.625 |
| 7 | 21 | 1.615384615 |
| 8 | 34 | 1.619047619 |
| 9 | 55 | 1.617647059 |
| 10 | 89 | 1.618181818 |
| 11 | 144 | 1.617977528 |
| 12 | 233 | 1.618055556 |
| 13 | 377 | 1.618025751 |
| 14 | 610 | 1.618037135 |
| 15 | 987 | 1.618032787 |
| 16 | 1597 | 1.618034448 |
| 17 | 2584 | 1.618033813 |
| 18 | 4181 | 1.618034056 |
| 19 | 6765 | 1.618033963 |
| 20 | 10946 | 1.618033999 |
| 21 | 17711 | 1.618033985 |
| 22 | 28657 | 1.61803399 |
| 23 | 46368 | 1.618033988 |
| 24 | 75025 | 1.618033989 |
| 25 | 121393 | 1.618033989 |
| 26 | 196418 | 1.618033989 |
| 27 | 317811 | 1.618033989 |
| 28 | 514229 | 1.618033989 |
| 29 | 832040 | 1.618033989 |
| 30 | 1346269 | 1.618033989 |
x
The ratio seems to pretty quickly converge to a number that is approximately 1.618033989.
What this means is that, before too long, .
Because , this means that the Fibonacci numbers appear to increase exponentially, with a multiplication factor of about 1.618033989.
What’s going on?
 Can we give a reason why the Fibonacci numbers increase exponentially, other than numerical evidence from a table of values?
Can we give a reason why the Fibonacci numbers increase exponentially, other than numerical evidence from a table of values?
And where does that strange number 1.618033989 come from?
Everything we know, and can know, about the Fibonacci numbers comes from the recurrence relation (1).
We are interested in the ratio so let’s divide the recurrence relation (1) by
to get:
x
…………………………………………(2)
x
In terms of the name for the ratio
, (2) says:
x
………………………………………..(3)
x
If – and for now it is just an “if” – conveges to some number
then the difference between
becomes very small as
increases, so
must satisfy:
x
x
………………………………………………(4)
x
x
Multiplying this through by gives a quadratic equation for
:
x
…………………………………………….(5)
x
The roots of this quadratic equation are:
x
.
x
This looks promising! It seems the mysterious 1.618033989 is just
In other words, it’s looking like for large
.
Can we prove it?
We have shown that IF converges to a number then that number must be
. The ratio cannot converge to the other root of the quadratic because the Fibonacci numbers increase with
.
What we have not yet shown is that, in fact, .
How can we do that?
One way is to examine the error and try to show that the error converges to 0 as
increases.
All we know about come from (3) and (4).
So:
That is, taking the size of the error:
x
x
Because the Fibonacci numbers increase we know that , so:
x
…………..(6)
x
Because , (6) tells us that the error
.
In other words, the ratio as the numerical evidence strongly suggested.
Numerical methods and numerical analysis
Numerical methods and numerical analysis
Numerical methods are what we use to get numerical information about an equation or a situation where we do not – yet – understand the algebra.
Numerical analysis is careful analysis of the errors to show that some quantity does, in fact, converge.
Numerical methods give us supporting evidence.
Numerical analysis shows this supporting evidence is, in fact, correct.