Republic of Mathematics blog

The axis of symmetry of a quadratic function

Posted by: Gary Ernest Davis on: November 27, 2010

In: Uncategorized
1 Comment

Jim Tanton (@jamestanton ) Tweeted:

“x=-b/(2a) ugh!For y=3x^2-12x+7 factor first 2 terms y=3x(x-4)+7 See x=0 & x=4 give same output. Axis of symm must be x=2.Try this on y=ax^2+bx+c!”

This, in my experience, is a simple yet typical example of Jim’s thinking: highly relational and generally avoiding instrumental procedures.

In this post I simply want to elaborate on this idea.

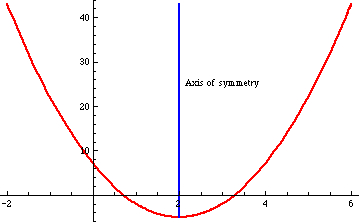
A quadratic polynomial function $p(x)=ax^2+bx+c$ is symmetric about some line $x= \textrm{ constant}$ . Also, $p(x)$ is either entirely increasing, or entirely decreasing, to the left of the line of symmetry, and to the right of the line of symmetry it is doing the opposite:

Students are often – usually? – taught that the axis of symmetry is $x=-\frac{b}{2a}$ without any rhyme or reason.

Knowing this is “knowledge that” something is so, without any relational understanding of why: students can “know that” without “knowing why”. Richard Skemp elaborated on the differences between relational and instrumental understanding, and his article is worth reading (again) in this context.

Let’s look in more detail at Jim Tanton’s example, above.

The quadratic polynomial $p(x)= 3x^2-12x+7$ can be written as $p(x)=3x(x-4)+7$ . This simply results from the common factor of “ $3x$ ” in $3x^2=3x\times x$ and $-12x = 3x\times (-4)$ .

We can make the term $3x(x-4)$ equal to 0 in two ways: by making $x=0$ and by making $x=4$ .

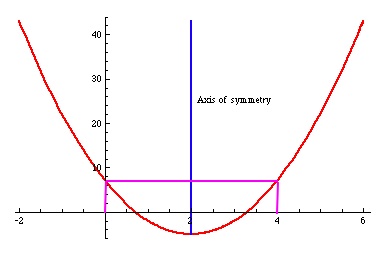
For both these values, $x=0 \textrm{ and } x=4$ , we have $p(x)=7$ .

In other words, at $x=0 \textrm{ and } x= 4$ the function $p(x)$ takes the same value (namely, 7).

Because $p(x)$ is symmetric about some line $x= \textrm{ constant}$ , and decreasing/increasing differently on opposite sides of the line of symmetry,Â this axis of symmetry occurs midway between $x=0 \textrm{ and } x=4$ , that is, at $x=2$ :

Let’s take another example: the quadratic polynomial $p(x)=5x^2-4x-2$ .

Using Jim Tanton’s idea we factor $5x$ from the first two terms to get $p(x)=5x(x-\frac{4}{5}x)-2$ .

From this we see, as before, that $p(0)=-2 = p(\frac{4}{5})$ .

Again, because the quadratic polynomial is symmetric about a vertical line and decreasing/increasing differently each side of that line, the line of symmetry is $x=\frac{0+\frac{4}{5}}{2}=\frac{2}{5}$ .

Applying this idea to a general polynomial $p(x)=ax^2+bx+c=ax(x+\frac{b}{a})+c$ , where $a\neq 0$ we see that $p(0)=p(-\frac{b}{a})=c$ so the axis of symmetry is midway between $0 \textrm{ and } -\frac{b}{a}$ , that is at $x=-\frac{b}{2a}$

Richard Skemp

Instrumental “understanding” involves being able to follow a set of instructions without knowing why you are doing so.

Relational understanding involves understanding the relationship of one thing to another to help guide you to a correct conclusion.

As Richard Skemp points out, relational understanding sometimes takes a little more mental effort to get what is going on, but pays off in terms of both flexibility, and a feeling of genuine understanding, stemming from seeing the relationship between various things.

Should students memorize the quadratic formula?

Posted by: Gary Ernest Davis on: November 26, 2010

In: Uncategorized
10 Comments

Recently, Dan Meyer @ddmeyer Tweeted:

“@MmeVeilleux Sorry if that’s a letdown. I wouldn’t have a kid memorize the quadratic formula.”

So, the question this raises for me, and that I want to address in this post is: should teachers require students to memorize the quadratic formula?

x

The quadratic formula

A quadratic polynomial is one of the form $p(x)=ax^2+bx+c$ where the $a, b, c$ are real numbers and where $a\neq 0$ .

Examples are $x^2-1, 2x^2+7x-2, -x^2+\sqrt{2}+3$ .

Quadratic polynomials have graphs, as functions of the variable $x$ and to plot quadratic polynomials we need to know whether the graph crosses the $x$ -axis and if so, where.

In other words, we need to find the solutions, if any, to the quadratic equation $ax^2+bx+c=0$ .

A usual method for solving this quadratic equation goes back to Diophantus and is known as “completing the square” and leads us to the formula

$x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$

The idea is a very simple one, yet in my experience, even mathematics, science and engineering undergraduates, let alone high school students, can get bogged down in the algebraic manipulations that are involved in the general case of completing the square.

The cognitive load is just too high for many students.

This is probably why many mathematics teachers want students to “memorize” the formula and just apply it to specific quadratic equations.

What might be less than helpful in this approach of rote memorization?

Rote memorization

This is about a useless a technique as was ever thought of for educational purposes, yet it is trotted out often by teachers: “Why won’t they just rote memorize it?”

What this concern betrays, in my experience, is a lack of understanding of human memory systems – the aspect of brain functioning that is critical to education.

Hermann Ebbinghaus

The definitive study on rote memorization was done by Hermann Ebbinghaus and published in 1885. He studied the rate of learning and forgetting of nonsense syllables by himself, and formulated his law of forgetting in which an exponential decay describes the rate at which rote memorized information is lost.

One might argue that mathematical terminology, facts, procedures, and formulas are not nonsense syllables, having a great deal of logical structure.

This argument misses the point that to many students the mathematics they are expected to remember appears to them to be more or less nonsense syllables, devoid of any real meaning.

Mathematical experience is not, for these students, something that they can fit into a broader scheme of related memories.

As a result their knowledge decays exponentially and, as experience shows, with a quite small half-life.

Rote memorization decays exponentially and we can only safely rely on recall of something rote-memorized if it is continually practiced and refreshed.

Permastore memory

Harry P. Bahrick

Harry Bahrick studies very long term memory.

Here’s what he had to say about long term memory and education in the Oxford Handbook of Memory:

“Educators have emphasized the immediate achievements of students, paying little attention to the effects of their instruction on long-term retention of content.”

His research has unearthed a number of important issues:

Instructional techniques that favor rapid learning may yield poor retention.
Spaced rehearsals can dramatically enhance accessibility of even marginal content for long periods of time.
Content that is not frequently accessed (e.g. mathematics) could remain accessible by implementing brief appropriately spaced retrieval practice sessions.

The major lessons for mathematics teachers that result from Bahrick’s research are thatÂ focusing on rapid achievement is likely to inhibit longer term retention. Practicing recall of earlier learned material at spaced intervals can dramatically increase longer term recall.

Schema

The idea of a mental scheme is that newly learned facts, procedures and other knowledge should fit into a pre-existing background of similar knowledge.

We learn things best, most flexibly, and for a longer time, if we relate them to things we already know.

This applies, in particular, to the quadratic formula: as a learned fact or procedure it is learned best with greatest chance of long term recall, and appropriate use in context, if it is learned in a way that relates to prior knowledge.

Theory of quadratic equations

The quadratic “formula” is really a theory of quadratic equations. It tells us under what conditions a quadratic equation has a solution, and when those conditions hold, it tells us what he solutions are.

My experience of working with high school students and undergraduates in several countries over many years is that few, if any, students are exposed to this simple, beautiful, and entirely memorable theory. Some of these students go on to become teachers of mathematics without ever having understood that the quadratic formula is really a theory of solutions of quadratic equations.

What is this theory?

It says that a quadratic equation $ax^2+bc+c=0$ , where that $a, b, c$ are real numbers and $a\neq 0$ , has real number solutions exactly when $b^2\geq 4ac$ . In this case the solutions are $x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$ .

So we can tell right off that the equation $x^2-x-1=0$ has real number solutions because $(-1)^2=1 > -4=4\times 1\times (-1)$ .

Equally we can tell that the equation $x^2-x+1=0$ has no real solutions because $(-1)^2=1 < 4=4\times 1\times 1$ .

How “should” a student solve quadratic equations?

I don’t know any “right” way to do this. Bearing in mind the difficulties with rote memorization, and problems with longer-term memory if a teacher focuses on rapid achievement, I would personally get students to spend time solving individual equations by completing the square.

For example, to discover if we canÂ find real number solutions to the quadratic equationÂ $5x^2-4x-2-0$ , and, if so, to find what those solutions are, we can proceed as follows:

Divide by the coefficient of $x^2$ . This gives us the equation $x^2-\frac{4}{5}x-\frac{2}{5}=0$
Move the constant term to the other side of the equation: $x^2-\frac{4}{5}x=\frac{2}{5}$
Add as much to both sides of the equation as is needed to make the left side a perfect square: $x^2-\frac{4}{5}x+\frac{16}{100}=\frac{2}{5}+\frac{16}{100}$
Re-write the equation as a perfect square equals a constant: $(x-\frac{4}{10})^2=\frac{14}{25}$
Recognize there are solutions if the right side of this equation is not negative: $\frac{14}{25}>0$
Take square roots: $x-\frac{4}{10}=\pm\sqrt{\frac{14}{25}}$ (remembering that a number and its negative square to the same result)
Rearrange to find the solutions: $x=\frac{4}{10}\pm\sqrt{\frac{14}{25}}$
Make things a little simpler if possible: $x=\frac{2}{5}\pm\frac{\sqrt{14}}{5}$

Sure, this is longer than applying the quadratic formula.

It takes longer to do more examples like this.

Achievement, as measured by writing down solutions of quadratics, is slower.

When students have a facility for this method, when at spaced intervals they are asked to recall how to do it, when they can recall this method longer term, then and only then are they ready for the quadratic formula.

In short, I am in agreement with Dan Meyer in that, in the beginning, I would not get students to (rote) memorize the quadratic formula.

Instead, I would focus on a clever method – completing the square – to figure if a quadratic equation even has solutions, and then use that to find the solutions.

Only after much practice of solving specific quadratic equations by completing the square, and by having spaced recall of that method, would I then introduce the general case with coefficients $a, b, c$ in place of specific numbers.

This, in my view, is the sort of thinking we want to instill in mathematical practice.

Postscript

Just after writing this post I noticed Jim Tanton’s (@jamestanton) excellent Guide to Everything Quadratic.

Jim also tweets: “ax^2 + bx + c = 0: Multiply by 4a to get 4a^2x^2+4abx+4ac=0. This is: (2ax+b)^2 – b^2 + 4ac = 0 and solve easily for x. What do you get?”

So let’s apply Jim’s method to the quadratic equation $5x^2-4x-2=0$ :