What constructivist mathematics is not …
Posted by: Gary Ernest Davis on: November 5, 2010
Over at Parenting is Political, @northTOmom has written a recent post:Â THIS MATH DEPRESSES ME.
Part of what concerns @northTOmom is what she identifies as “constructivist” math.
Here is what she writes:
 “So why do I object to constructivist math? One reason is that it is, by-design, non-incremental or “spiral”: its textbooks jump around from topic to topic, never staying on a subject long enough to allow for deep understanding or competence. I also dislike reform math because it frowns upon direct instruction. Since constructivist math teachers believe children can “construct” or “discover” mathematical truths and come up with their own algorithms to solve problems, they offer students minimal guidance, and are not averse to putting the cart before the horse: e.g., assigning algebra-type problems before teaching the tools of algebra, or asking kids to divide or multiply by decimals or fractions without having first taught them how decimals and fractions work.”
“So why do I object to constructivist math? One reason is that it is, by-design, non-incremental or “spiral”: its textbooks jump around from topic to topic, never staying on a subject long enough to allow for deep understanding or competence. I also dislike reform math because it frowns upon direct instruction. Since constructivist math teachers believe children can “construct” or “discover” mathematical truths and come up with their own algorithms to solve problems, they offer students minimal guidance, and are not averse to putting the cart before the horse: e.g., assigning algebra-type problems before teaching the tools of algebra, or asking kids to divide or multiply by decimals or fractions without having first taught them how decimals and fractions work.”
I was surprised to read this because it’s not what I understand constructivist theory applied to mathematics teaching to be. In fact so far as I know, constructivist theory is a theory of learning and says little to nothing about teaching. It is a theory of how people learn, which might be very useful to teachers, but does not imply “discovery” learning.
As I recall, one of the fathers of constructivist thought, Jean Piaget remarked that it is absurd to imagine children can, or will, discover large parts of mathematics for themselves: there’s just simply too much of it, most of it thought of by mature adults.
It seems to me that part of the problem here is one of semantics compounded by the U.S. Bill of rights: every U.S. citizen feels free to use whatever words they choose in whatever way they choose to use them, without reference to previous thinkers and scholars. Such a polyglot leads to gross misunderstanding in communication, and eventually to misinformed practice as @northTOmom observes.
Constructivism in mathematics classes is not about letting children “discover” mathematics. What it is about, to a first approximation is students reflecting on their actions.
Piaget used the term “reflective abstraction”; the power of generalization and abstraction from reflecting on one’s actions. We see this for example when young children count – to 10, for example – and we ask them afterward: “When you were counting those 10 crackers, what if I gave you 2 more? How many would you have then?”
If the child already has experience in reflecting on their counting they may be able to solve this problem by imagining the objects in their mind. If not, they will probably look for 2 more crackers and count again using the real crackers.
Ernst von Glasersfeld points out that Piaget’s use of reflective abstraction as a cornerstone of a constructivist theory of learning was not original with him. Already in 1690 John Locke had observed:
“the ideas it affords being such only as the mind gets by reflecting on its own operations within itself. (Locke, 1690, Book II, 4)
So, if your child’s mathematics teacher calls themselves a constructivist teacher, ask what that means. If they mention discovery learning, and do not mention reflective abstraction as a core idea, then they either do not understand constructivism, or do not understand mathematics, or both.
Piaget’s ideas spoke to a truly experimental view of knowledge: how is that we obtain new knowledge as we make our way in the world?
Reflective abstraction is a key part of his theory of how we do that. In and of itself it has little to do with teaching, yet because it is about learning a wise teacher will look for ways to encourage students to reflect on their experiences, and to abstract and generalize from them.
Reading
Constructivism in Education, Leslie P. Steffe (Editor), Jerry Gale (Editor), Routledge (1995). ISBN: 978-0805810967
Radical Constructivism in Action: Building on the Pioneering Work of Ernst von Glasersfeld, Leslie P. Steffe (Editor), Patrick W. Thompson (Editor). Routledge (2000). ISBN: 978-0750709897
Postscript
Raymond Johnson alerted me to Lee V. Stiff’s 2001 article “Constructivist Mathematics and Unicorns“. In this article Lee gives a decent summary of constructivism and points out how opponents of certain progressive classroom practices in mathematics use the term “constructivist” pejoratively.
Many thanks Raymond for this link.
High stakes tests, mathematics word problems, & second-language learners
Posted by: Gary Ernest Davis on: November 4, 2010
 High stakes tests and word problems
High stakes tests and word problems
It is increasingly expected, and in most U.S. states required by law, that students will learn and achieve at grade-level standards if they are to graduate from high school.
In mathematics, the tests used to measure whether students meet grade-level standards often involve word problems set in contexts considered to reflect experiences and knowledge readily available to the students.
Limitations of working memory
A problem solver needs to recall domain-specific knowledge in long-term memory relevant to the problem situation, hold it in working memory, and simultaneously work on the solution to the problem.
Limited amount of information can be stored and processed in working memory.
This creates a cognitive load.
Kalyuga, S., Ayres, P., Chandler, P., & Sweller, J. (2003). The expert reversal effect. Educational Psychology, 38, 23–31.
English language learners & mathematics teachers
Teachers need to:
Nieto, S. (2004). Affirming diversity: The sociopolitical context of multicultural education (4th ed.) Boston: Pearson Education.
A genuine, and typical, word problem
The Laundry Problem
Sandy’s family does its laundry at a coin-operated laundromat. It costs $1.25 per load to use the washing machines and 25¢ per load to use the dryers for 10 minutes. Sandy’s family has 5 loads of laundry to do and each load will need to be in a dryer for 30 minutes. Which expression will give Sandy’s family the total cost of doing these loads of laundry?
A. ($1.25 + $0.25) × 3 × 5
B. [$1.25 + (3 × $0.25)] × 5
C. [(3 × $1.25) + $0.25] × 5
D. 3 × ($1.25 + $0.25) × 5
From the 2002 Washington Assessment of Student Learning (WASL) for Mathematics.
 A reasonable problem for seventh graders?
A reasonable problem for seventh graders?
About half (49.9%) of more than 70,000 students who took the test were unable to manage the cognitive demands of this problem to select the correct answer. (Choice B)
About one-third (33.9%) of the students linked the information within the second sentence (Choices A and D) instead of linking information from the second sentence with information from the third sentence.
Students’ lack of prior experience with laundromats could be expected to impact their success rate.
What is really being tested by this problem?
Linguistic challenges
Use of the pronoun it in the sentence, “It costs $1.25 per load to use the washing machines and 25¢ per load to use the dryers for 10 min,â€
Compounding & cognitive demands; “and 25¢ per load,†links the “25¢ per load†with the washing machine load until the end of the sentence -prior experience with laundromats signals the reader to interpret the sentence differently.
Spanish speakers, for example, may interpret the words to do as make.
Knowledge assumptions: a student may be familiar with wash clothes in English but not with the word laundry, and students whose families do laundry at home may not understand the word laundromat.
Load is usually first learned as a verb and when second-language learners read the word expression, they may, for example, think about what someone’s face looks like.
For many ESL students, English first names are not easily recognized; “What is a Sandy?â€
Another one for you to think about …
The Soccer Problem
Jorge’s town has a soccer league. He helped sign up players for the different divisions. The table below shows the total number of players who signed up this year.
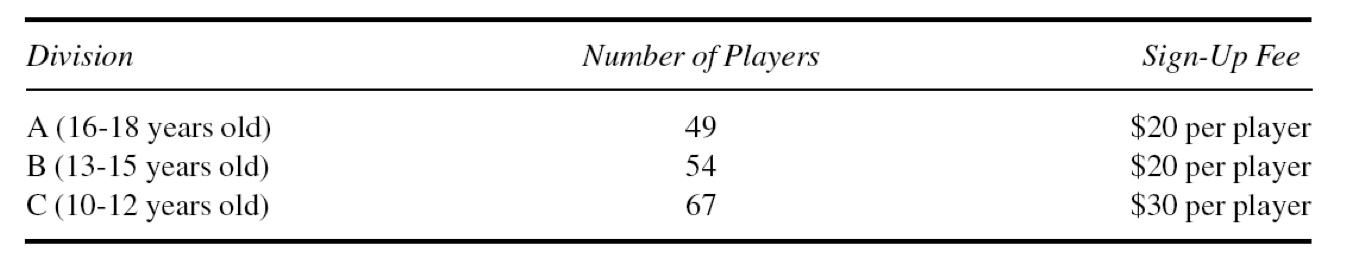 Jorge needs to figure out the total amount of money from sign-up fees.
Jorge needs to figure out the total amount of money from sign-up fees.
Which of the following expressions will give him the correct amount?
A. (49 + 54) × $20 + (67 × $30)
B. (49 × $30) + (54 × $20) + (67 × $30)
C. (49 + 54 × $20) + (67 × $30)
D. (49 + 54 + 67) × ($20 + $30)
Underestimating what students know
How students use everyday knowledge to answer mathematics test items that use “realistic†contexts to frame the test question.
Cooper, B., & Dunne, M. (1998). Anyone for tennis? Social class differences in children’s responses to national curriculum mathematics testing. Sociological Review, 46, 115–148.1998;
Lamon, S. (2003). Beyond constructivism: An improved fitness metaphor for the acquisition of mathematical knowledge. In R. Lesh & H. M. Doerr (Eds.), Beyond constructivism: Models and modeling perspectives on mathematics problem solving, learning, and teaching (pp. 435–447). Mahwah, NJ: Lawrence Erlbaum
Underestimation of students’ actual capacities with respect to mathematics may occur as a result of second-language learners’ lack of familiarity with the context of a problem.
When a teacher and students do not share the same language, previous experiences, or culturally based assumptions about what it means to teach and learn mathematics, the teacher’s resulting mental model of student learning may have little to do with the students’ actual understanding and construction of mathematical concepts and processes.
A check list for mathematics teachers
Reference
Campbell, A. E., Adams, V.M. & Davis, G.E. (2007) Cognitive demands and second language learners: A framework for analyzing mathematics instructional contexts. Mathematical Thinking & Learning, 9(1), 3-30