The value of cos(pi/5)

Posted by: Gary Ernest Davis on: December 3, 2010

0 Shares

@capedsam Tweeted: “Can you find an exact value for cos(pi/5)? “

It is actually fairly easy to find an exact value for $\cos (\frac{\pi}{5})$ by following a path through the complex numbers.

To do this we use Euler’s identity $e^{ix}=\cos (x) + \sin (x)$ for all real numbers $x$ .

Jim Tanton (@jamestanton) has a very nice introduction to Euler’s identity here.

As shorthand let’s write 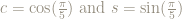$c=\cos (\frac{\pi}{5}) \textrm{ and } s=\sin (\frac{\pi}{5})$ .

From Euler’s identity we know that:

$e^{i\frac{\pi}{5}}=\cos (\frac{\pi}{5}) + \sin (\frac{\pi}{5}) =(c+is)^5$ ………(1)

We raise both sides of equation (1) to the 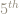$5^{th}$ power:

$(e^{i\frac{\pi}{5}})^5=(c+is)^5$ ……………………….(2)

The left hand side of equation (2) is $(e^{i\frac{\pi}{5}})^5=e^{i\pi} =-1$ .

The right hand side of equation (2) is, by the binomial theorem:

$(c+is)^5 = c^5+5ic^4s-10c^3s^2-10ic^2s^3+5cs^4+is^5$ Â ……..(3)

We separate the expression in (3) into real and imaginary parts, and equate the real part to -1, and the imaginary part to 0:

$c^5-10c^3s^2+5cs^4=-1$ ……………………………..(4)

$5c^4s-10c^2s^3+s^5=0$ ……………………………….(5)

We can substitute $1-s^2 \textrm{ for } c^2$ in (5) to get:

$5(1-s^2)^2s-10(1-s^2)s^3+s^5=0$ ………….(6)

Expanding (6) and dividing through by $s$ we get:

$16s^4-20s^2+5=0$ ………………………………………(7)

This is a quadratic equation for $s^2$ with roots $\frac{5+\sqrt{5}}{8}\textrm{ and } \frac{5-\sqrt{5}}{8}$

This gives 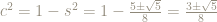$c^2=1-s^2 = 1-\frac{5\pm\sqrt{5}}{8} = \frac{3\pm\sqrt{5}}{8}$

Only one of these two roots can be equal to 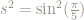$s^2=\sin ^2(\frac{\pi}{5})$ .

Which one?

We know $\sin (\frac{\pi}{5}) < \sin (\frac{\pi}{4}) =\frac{\sqrt{2}}{2}$ so $s^2 < \frac{2}{4}=\frac{1}{2}$ .

Therefore, $s^2= \frac{5-\sqrt{5}}{8}$ so $c^2=\frac{3+\sqrt{5}}{8}$ which means, since $\cos (\frac{\pi}{5})>0$ , that:

$cos (\frac{\pi}{5})=\sqrt{\frac{3+\sqrt{5}}{8}}$ .

One can also get the quadratic equation for $s^2$ from the multiple angle formulas (if one can remember them!).

Republic of Mathematics blog